What you’ll need:
- A pencil
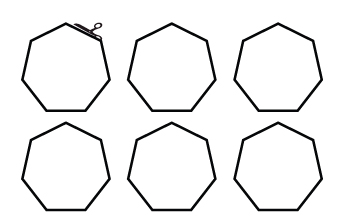
- The heptagon template
- Paper, old greeting cards, bristol board or thin cardboard (e.g., from a cereal box)
- Scissors
- Clear tape
- Markers, pencil crayons or paint
Investigate hyperbolic geometry with curvy coral shapes.
Humans have created a world of straight lines. The rooms we live in, the pages we write on and the gridlike arrangement of our streets all reflect conventional, straight-lined Euclidean geometry.
In contrast, the fruits, vegetables, trees and coral found in the natural world reflect spherical and hyperbolic geometry. Spherical geometry is the geometry of regular spheres, which are positive curves, while hyperbolic geometry refers to negative curves or saddle shapes. The ruffled edges of kale leaves and coral are both examples of hyperbolic geometry.
In 1997, mathematician Daina Taimina found a simple, unexpected way to demonstrate hyperbolic geometry in three dimensions: crochet!
Taimina realized that she could easily make a negative curve by adding extra stitches at regular intervals in her crocheted pieces. Her work later inspired Australian sisters Margaret and Christine Wertheim to begin Crochet Coral Reef, a global community initiative that celebrates hyperbolic geometry, citizen science, environmentalism and, of course, coral reefs.
On a flat Euclidean surface, it’s possible to draw two parallel lines that remain equally distant from each other. On a spherical or hyperbolic surface, the two lines will curve together and apart from each other—they can never be parallel.
Try drawing parallel lines on your hyperbolic heptagon creation. What do you notice about the lines?